Vijay Sonnad (15-FS-005)
Abstract
Realistic calculations for quantum many-body systems pertaining to the properties of microscopic systems made of a large number of interacting particles require innovative methods and large-scale computational approaches. In theoretical physics, the problem of mathematically expressing values of a many-particle system is usually so difficult that it requires approximate methods, the most common of which is expansion of basis functions that obey the boundary conditions of the problem. The computational effort needed in such problems can be much reduced by making use of symmetry-adapted basis functions, which exploit the symmetry of the system under investigation. We explored a mathematical approach based on the unitary group approach to atomic electronic-structure calculations and its graphical representation, which has been highly successful in quantum molecular structure calculations. The first part of the project involved solving for a synthetic Hamiltonian and comparing traditional solution methods with approaches that use group-theoretical structures. The second part focused on developing an understanding of the various components of the unitary group approach compared to the traditional Racah-algebra-based technique and implementing this approach for relatively simple calculations in atomic and nuclear structure. The synthetic Hamiltonian was selected for its relevance to deformed nuclear systems and its dynamical-symmetry; solutions were obtained with a state-of-the art shell-model code. We explored the issues of symmetry adapted truncation schemes to overcome size constraints, concluding that, in this context, the symplectic group in three dimensions, Sp(3,R), is more promising than a unitary group approach. In extending the unitary group approach to atomic and nuclear structure calculations it is necessary to deal with spin-dependent Hamiltonians with many body interactions, and multi-shell bases satisfying additional symmetries. Our finding is that each one of these introduces significant additional complications to the base unitary group approach; in combination, they demand very formidable machinery with highly involved derivations. We concluded that, in spite of the elegance and conceptual clarity of the unitary group approach, it does not, at this time, offer a simpler alternative to Racah-algebra-based approaches for atomic and nuclear structure calculations.
Background and Research Objectives
Codes for quantum many-body structure calculations in atomic, nuclear, and molecular physics play important roles and are among the most heavily used in their respective fields. The typical approach is to discretize the Hamiltonian by expanding the wave function in a set of basis functions resulting in matrix eigenproblems. Basis functions, which are adapted to the symmetry inherent in the problem, can result in significantly smaller matrices often leading to dramatic savings in computational effort. The traditional approach with symmetry adapted bases utilizes Racah algebra, which is highly effective but is notoriously difficult and requires a customized approach for each class of problems.
We investigated the feasibility of using the unitary group approach as a simpler alternative to Racah algebra with symmetry adapted bases. The unitary group approach has been highly successful in molecular structure calculations but has not been used as an alternative to Racah algebra in atomic or nuclear structure calculations. The unitary group approach is based on two fundamental concepts: the first is the re-expression of the Hamiltonian in terms of generators of the unitary group; the second is the use of Gelfand Tsetlin bases to discretize the Hamiltonian.The Gelfand Tsetlin bases are not adapted to any physical symmetry and are thus not efficient for problems possessing symmetry. Symmetry-adapted bases are obtained as linear combinations of Gelfand Tsetlin bases, which are eigenvectors of the Casimir operators of the required symmetry group discretized in the Gelfand Tsetlin bases; these linear combination of Gelfand Tsetlin bases are the ones that are used in the computations.
The unitary group approach provides a conceptually unified framework to solve structure problems which is, in principle, equivalent to the Racah algebra approach. The unitary group approach however has considerable potential as a general purpose technique for quantum structure calculations: Hamiltonians from entirely different fields are cast into a set of “standard” operators, and symmetry-adapted basis functions from different fields are cast into a set of “standard” basis functions. This opens up the very attractive possibility of a single, standardized computational approach to structure problems in a variety of different fields.
Our objective was to fully understand developments in the unitary group approach, use it to solve relatively simple structure problems in atomic and nuclear physics, and then compare the unitary group approach against the Racah algebra approach. Our focus was on determining whether the unitary group approach proved simpler than Racah algebra to understand, derive, and implement with minimal sacrifice in computational efficiency. The focus on simplicity was not based merely on aesthetic considerations. The efficiency of this important class of codes on graphics processing units could be greatly enhanced if a much simpler implementation were available. An approach developed previously uses a relatively simple determinantal approach with low penalty in computational efficiency over Racah algebra for many problems of practical interest.1 Having evidence that simpler approaches could be competitive with Racah algebra provided impetus for the investigation.
For reasons mentioned below, we were unable to code the various components required to solve problems with the unitary group approach in the time allotted for this study. The unitary group approach is most directly suited to single shell, spin-free Hamiltonians in spin-orbit coupling, which explains its wide acceptance in molecular structure calculations. However with spin-dependent Hamiltonians, the formation of matrix elements between Gelfand bases requires very formidable machinery with highly involved derivations (especially for multi-shell problems). The conceptual unity of the unitary group approach framework is not enough to overcome these difficulties, and we concluded that, at the present time, the unitary group approach does not offer a simpler alternative to Racah-algebra-based approaches.
Scientific Approach and Accomplishments
We divided this project into two main subprojects: (1) solve a problem in nuclear structure with a specially constructed synthetic Hamiltonian and also compare traditional solution methods with approaches that use group-theoretical structures; and (2) understand the various components of the unitary group approach with the goal of implementing it in a code.
To address the first problem, we hired a nuclear physics graduate student from Louisiana State University in Baton Rouge, as a summer intern in 2015. He applied a family of two-term, symmetry-adapted Hamiltonians to C-12 in its full, no-core, shell-model space using the BIGSTICK shell-model code.2
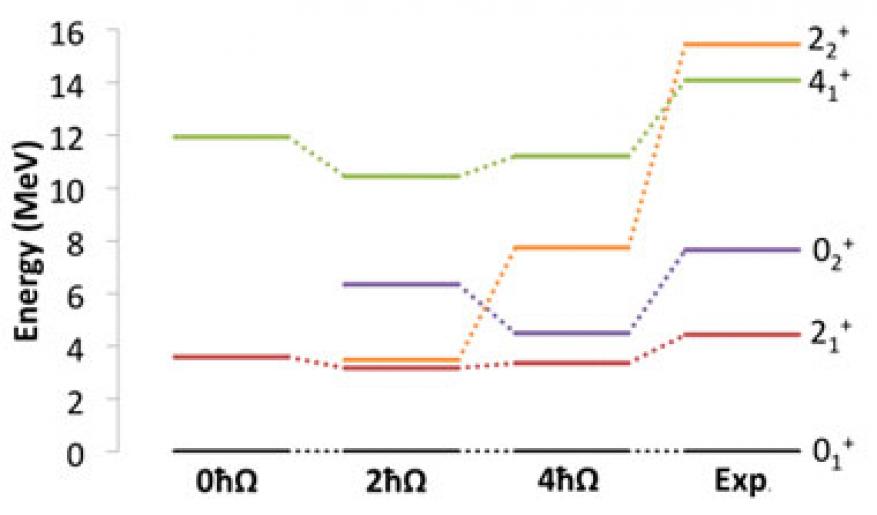
The first term, the harmonic oscillator Hamiltonian, confined the protons and neutrons inside a harmonic oscillator potential and reproduced the expected shell structure. The second term, the mass quadrupole moment operator dotted with itself, represented the interaction of one particle with the total quadrupole moment of the system and came with an adjustable parameter that allowed us to change the overall spacing between states on the energy spectrum so that they better reproduced the experiment. The initial results, up through Nmax = 4, suggest that this effective Hamiltonian can reproduce the main features of the carbon-12 spectrum, including the ordering of the lowest 0+, 2+, and 4+ states, as shown in Figure 1.
A major hurdle that modern (multi-shell) shell-model calculations have to overcome are large model spaces, which increase dramatically with increasing particle numbers. This also applies to nuclear reaction calculations which use nuclear-structure information as input. Symmetry-adapted truncation schemes have been suggested to overcome the difficulties, with one promising approach making use of the symplectic group in three dimensions, Sp(3,R); however, ingredients are required to carry out more complete calculations which employ modern nuclear Hamiltonians.4 Also rigorous application of the Wigner-Eckhart theorem makes it possible to dramatically reduce the number of matrix elements that need to be calculated, a fact that is potentially significant, as calculations (especially for reactions) are limited by the number of matrix elements that can be stored. For the SU(3) group, all relevant ingredients are currently available, but for the symplectic group, the required coupling and re-coupling coefficients would have to be derived.
The second subproject was initiated by studying the literature starting with the early days of the unitary group approach. While there are excellent introductions to the foundations of this approach, subsequent developments that allow the application of this approach to spin-dependent Hamiltonians with multi-shell Gelfand Tsetlin bases are limited to highly specialized research papers that require advanced knowledge in a variety of techniques. We developed an overview of the unitary group approach that may help others understand the method more quickly.5 In the following paragraphs, we briefly outline the major steps in the unitary group approach to explain the increase in difficulty with multi-shell bases and spin-dependent Hamiltonians.
A quantum mechanical Hamiltonian for structure calculations can be expressed in terms of creation–annihilation operators; a product of a creation and annihilation operator is termed a replacement operator. It is a profound insight that replacement operators satisfy the same commutation relations as the generators of the unitary group. The Gelfand Tsetlin bases are symmetry-adapted to the unitary group in that they form irreducible invariant subspaces under the action of the generators of the unitary group. They are specified in a highly abstract fashion by a triangular pattern of numbers, termed a Gelfand pattern, which represents a significant achievement in the theory of unitary groups and are a tour-de-force of compactness in the amount of information that is encoded into each pattern. An important development in the unitary group approach was the realization that it was not necessary to use the general Gelfand Tsetlin bases for electronic calculations; a simplified representation was obtained that used only 3n labels as compared to n(n+1)/2 labels for the general Gelfand Tsetlin bases.
The Gelfand Tsetlin bases used in unitary group approach are initially adapted to U(2n) where 2n is the total number of spin orbitals in the problem. A critical step in this approach breaks up U(2n) basis functions into products of orbital and spin functions (described schematically as U(2n) ⊃ U(n)⊗U(2) ). A spin-independent Hamiltonian can be expressed in terms of generators of U(n), and matrix elements are essentially formed with U(n) generators and U(n) adapted Gelfand Tsetlin bases, with the U(2) spin bases playing a secondary role. However spin-dependent Hamiltonians can only be expressed in terms of generators of U(2n). Calculating the matrix elements of U(2n) generators with U(n)⊗U(2) Gelfand Tsetlin bases is a very challenging problem and there are at least two separate approaches to solving the problem. The final expressions are difficult to obtain; the difficulty of the problem is evidenced by the fact that separate groups worked on each of these approaches for over a decade to get the final expressions.
The problem of multi-shell Gelfand Tsetlin bases is best explained for a two-shell problem and uses the decomposition U(n) ⊃ U(n1)⊗U(n2) where n = n1+n2, i.e, each basis is expressed as a sum of products of bases limited to each shell. Obtaining matrix elements between multi-shell bases is less difficult than the problem with spin. However the combination of a multi-shell basis with spin-dependent Hamiltonians along with multi-body generators results in fiercely complex expressions. It is also necessary to adapt the multi-shell Gelfand Tsetlin bases to the specific symmetry of the problem (typically achieved by calculating eigenvectors of Casimir operators corresponding to the symmetry group). The overall unitary group approach is more difficult to understand and derive than the Racah algebra approach (mostly because of very unfamiliar techniques in the derivations) and is not obviously easier to implement.
Impact on Mission
Our work exploring approaches for reducing matrix size in atomic and nuclear calculations supports the Laboratory's core competency in high-performance computing, simulation, and data science. In atomic physics, the benefit of a single unified approach across several codes and a path towards the development of newer, higher-accuracy codes would also be relevant to LLNL's core competency in nuclear, chemical, and isotopic science and technology.
Conclusion
The unitary group approach is an elegant framework that provides a conceptually unified foundation for quantum structure calculations. It is very well suited to spin-free calculations and has been widely used in molecular structure calculations. While it is certainly possible to extend the unitary group approach to solve structure problems in atomic and nuclear physics, it becomes dramatically more complex and is not a preferable alternative to existing methods that use Racah algebra. In examining the unitary group approach, it became evident that the underlying reason for the difficulty is that the action of different operators on the Gelfand Tsetlin bases are difficult to obtain. There have been several points in the history of the unitary group approach where it received powerful impetus due to important simplifications; it is our belief that such simplifications will continue, and this elegant method will live up to its promise over time.
While our study concluded that the unitary group approach is not a simpler alternative to traditional Racah-algebra-based approaches, it has provided insight into some useful group theoretical concepts, and this knowledge could prove important in other contexts. This study has also provided a strong foundation in the unitary group approach itself and there is every reason to believe that further research will yield valuable results. Our summer intern has continued to work towards his PhD and has made notable progress on other methods for nuclear structure calculations at Louisiana State University.
References
- Hill, E., “Calculation of unit tensor operators using a restricted set of Slater determinants." J. Quant. Spectrosc. Radiat. Transf. 140, 1 (2014). http://dx.doi.org/10.1016/j.jqsrt.2014.02.009
- Baker, R., et al., Smart bases and instructive interactions in large-scale quantum many-body systems. (2015). LLNL-POST-675543.
- Johnson, C. W., et al., “Factorization in large-scale many-body calculations.” Comput. Phys. Comm. 184(12), 2761 (2013). http://dx.doi.org/10.1016/j.cpc.2013.07.022
- Escher J., Towards large-scale multi-shell calculations with symmetry-adapted bases: Lessons from the symplectic shell model. Lawrence Livermore National Laboratory. (2016).
- Sonnad, V., et al., An informal overview of the unitary group approach. (2016). LLNL-MI-693025.
Publications and Presentations
- Baker, R., et al., Smart bases and instructive interactions in large-scale quantum many-body systems. (2015). LLNL-POST-675543.
- Sonnad, V., et al., An informal overview of the unitary group approach. (2016). LLNL-TR-694837.