Joseph T. McKeown (15-ERD-006)
Abstract
Rapid solidification is prevalent during laser-based additive manufacturing (AM), resulting in parts with non-equilibrium microstructures and unique properties. Understanding microstructure evolution during rapid solidification (RS) has been hindered by the inability to characterize these processes with in situ techniques due to the high solid–liquid interface velocities. This has limited the ability to develop accurate predictive models for rapid solidification. The goal of this project was to develop a framework that enables validation of a predictive mesoscale model for rapid solidification using time-resolved in situ characterization of microstructure evolution during RS. The approach employed dynamic transmission electron microscopy (DTEM) to capture the rapid solidification process with high temporal resolution (microsecond timescales) to provide critical input data to a phase-field model. Using a binary Ni-Cu alloy, excellent agreement between the results of experiments and simulations was obtained under rapid-solidification conditions, providing a framework for validation of the phase-field model. This project has laid the groundwork for future efforts to describe the conditions under which microstructure develops under AM conditions and provides a path for high-temperature in situ rapid-solidification experiments using a broader class of alloys for AM.
Background and Research Objectives
The production of complex metal and alloy components using additive manufacturing (AM) techniques represents a radical departure from conventional manufacturing processes. Qualification challenges exist for wide-ranging adoption of AM of metals, where concern is mainly focused on the quality of the additively manufactured material (King 2014; Mani et al. 2015). Components produced by AM often exhibit microstructures and properties that differ significantly from those of conventional cast and wrought alloy components, a result of the processing conditions that exist during AM.
Rapid-solidification (RS) conditions are prevalent during AM of metals and alloys (McKeown et al. 2016), an inherently nonequilibrium process. Microstructural spatial scales, metastable phase formation, compositional segregation, distribution of phases, and crystallographic texture are all factors that can vary greatly as the solid–liquid transformation front is driven further from equilibrium (Gerlach 1994). Understanding the microstructure evolution and kinetics of these far-from-equilibrium phase transformations at the requisite timescales is essential for developing validated predictive-modeling AM capabilities. In situ studies of RS can provide significant scientific and technological insight for AM processing.
Accessing the temporal regimes under which RS occurs with in situ characterization techniques has proved challenging experimentally. Experimental insight into microstructure evolution during RS has been based almost entirely on ex situ post-mortem analyses (Zimmermann et al. 1989; Gill and Kurz 1993; Kurz and Gillian 1994), where reported solidification-front velocities typically represent averages for the complete solid–liquid transformation (Kittl et al. 2000). This inability to monitor and characterize RS in situ is due to the extremely high solidification-front velocities (10-2 – 102 m/s) that result from large thermal gradients (105 – 107 K/m) and cooling rates (103 – 107 K/s) during RS (Kline and Leonard 2005; Kulovits et al. 2009; Zhong et al. 2009; Kulovits et al. 2011; McKeown et al. 2014). These extreme non-equilibrium conditions and the lack of experimental data severely limit the ability to accurately model RS and predict resultant microstructures.
The goal of this project was to develop a scientific basis and framework to relate materials (alloy systems) and laser-processing parameters to resultant RS microstructures using an approach that integrates ultrafast in situ characterization with mesoscale predictive-modeling capabilities. Direct in situ observations of the evolving microstructures during laser-induced RS were acquired using the dynamic transmission electron microscope (DTEM) (Campbell et al. 2014; LaGrange et al. 2015; McKeown et al. 2016) at Lawrence Livermore National Laboratory, providing accurate measurements of the solidification kinetics. This data served as critical input to validate phase-field simulations of RS at similar lengths and timescales, providing a science-based path toward prediction of non-equilibrium microstructures under the extreme conditions of laser melting that can be extended across materials systems for AM processes.
Scientific Approach and Accomplishments
The scientific approach employed experiments and simulations with similar spatial and temporal scales. Time-resolved in situ RS experiments were conducted in the DTEM using numerous alloy systems, with the major accomplishments obtained using Ni-Cu alloys. Phase-field simulations were conducted using the Laboratory’s Adaptive Mesh Phase Evolution (AMPE) phase-field code (Dorr et al. 2010; Fattebert et al. 2014) implemented on the Laboratory's massively parallel high-performance computing (HPC) capabilities.
Experimental
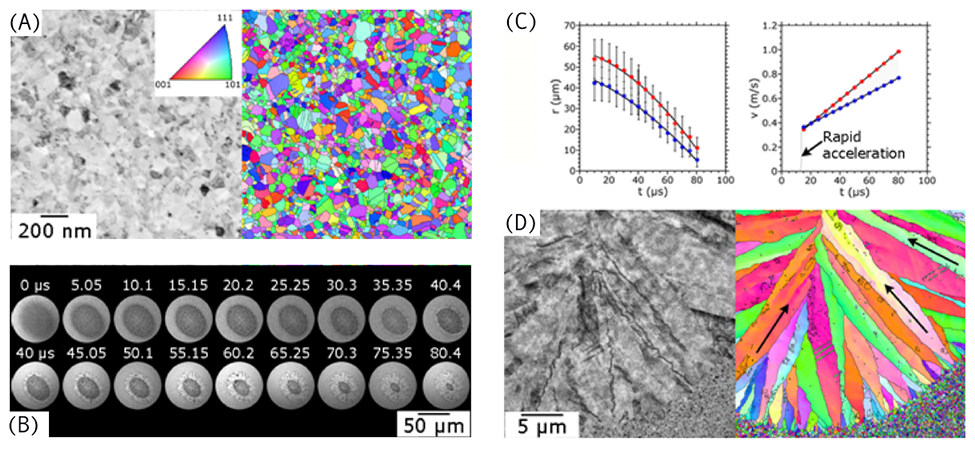
To perform in situ RS experiments in the DTEM, thin alloy films of a desired composition were deposited using DC magnetron sputtering onto 50-nm-thick bilayer substrates of amorphous silicon nitride (a-SiN) and amorphous aluminum oxide (a-Al2O3) layers. The alloy films were approximately 100 nm thick, with the a-Al2O3 layer located between the alloy film and the a-SiN layer. Figure 1 presents an overview of a representative experimental data set acquired from an alloy thin film with a composition of 45 atomic percent (at.%) nickel and 55 at.% Cu (Ni-55Cu).
The initial, as-deposited microstructure is shown in Figure 1(A), with a bright-field DTEM image (left) and an associated crystal-orientation map (right). The inset standard-orientation triangle provides the color-coded crystallographic orientations as they apply to the orientation maps in Figures 1(A) and 1(D). The average grain size of the as-deposited films was approximately 40 nm and there was no preferred crystallographic texture in the film.
Figure 1(B) presents a DTEM time-delay image series acquired during laser-induced RS. A 15-ns Gaussian-profile laser with a total deposited energy of approximately 43 µJ was used to melt regions of the alloy film; the elliptical melt pool is the central region of dark contrast in each of the 18 frames in Figure 1(B). The shapes of the melt pools were elliptical as a result of the 45° angle of incidence of the Gaussian laser pulse relative to the alloy film normal. Each of the 18 frames was acquired with a 50-ns electron pulse with a 5-µs interframe spacing. Each row of nine images in Figure 1(B) is a separate RS experiment performed on a fresh, unmelted region of the Ni-55Cu thin film. A full sequence of nine images spanned 40.4 µs. Further details of DTEM experimental parameters can be found in Campbell et al. (2014), LaGrange et al. (2015), and McKeown et al. (2016). The times indicated above each frame are the delays (in µs) between the peak of the Gaussian laser pulse that melted the film and the 50-ns electron pulse used to form the image. Details of the microstructure evolution can be discerned from the time-resolved DTEM images: the solid–liquid interface appears to be smooth, sharp, and morphologically planar during RS with a columnar growth morphology that resulted from the initial nanocrystalline state of the alloy film.
The solidification kinetics were measured directly from the time-delay images, and plots of the time evolution of the melt-pool dimensions (r) and solidification-front velocity (v) are provided in Figure 1(C). Quantitative measurements of the time evolution of the semi-major and semi-minor axes of the elliptical melt pool were performed using the ImageJ (Rasband 2018; Schneider et al. 2012) software platform. In the plots in Figure 1(C), the data in red and blue correspond to measurements acquired along the semi-major and semi-minor axes of the melt pool, respectively. Both axes decrease monotonically with time during RS, and the rate of change is higher along the semi-major axis. This is a consequence of the local curvature along the elliptical melt pool, leading to a higher rate of heat extraction along this axis. This also implies a range in the rate of change of the axis length; this is indicated by the gray shading in the plot, with maximum and minimum local curvature (and, hence, heat extraction) along the semi-major and semi-minor axes, respectively. The data acquired from each axis were fit to a second-order polynomial, shown as the line fit to the data in the plot in Figure 1(C).
The polynomial expressions were differentiated with respect to time to obtain linear expressions for the solidification-front velocity with time plotted along each axis. These velocities are also plotted in Figure 1(C), where the gray shading represents a range of velocities that depend on positions along the solid–liquid interface. It is evident from the plot that the solid–liquid interface accelerated as RS progressed, with an increase in velocity of approximately 0.35 m/s at 15.15 µs to velocities of approximately 1.0 m/s and ~0.7 m/s along the semi-major (red) and semi-minor (blue) axes at 80.4 µs, respectively. An initial rapid acceleration occurred prior to the steady-state columnar growth observed in the DTEM images of Figure 1(B), as indicated in the velocity plot of Figure 1(C). This rapid acceleration was not captured under these imaging conditions (McKeown et al. 2016).
Figure 1(D) shows a BF DTEM image (left) with the associated crystal-orientation map (right) acquired from the melt pool after RS, showing the columnar grains with the growth direction (indicated by the arrows in the orientation map) along the direction opposite of heat extraction. These columnar grains were approximately 5–10 µm long, 1–2 µm wide, and 100 nm thick, with certain grains occluded during growth (not all grains extend to the center of the melt pool). Again, there was no preferred crystallographic texture on the final, rapidly solidified microstructure.
In addition to the work with Ni–Cu, numerous alloy systems were investigated, including Al-based alloys such as Al–Si and Al–Cu, and titanium-based alloys such as Ti–Nb and Ti-6Al-4V. The Ti-based alloy systems have much higher melting temperatures and lower thermal conductivity (on the same order of magnitude with the substrate materials) than the Al- and Ni–Cu-based alloys systems. This condition leads to mechanical instability of the substrate at elevated temperatures because the heat extraction is no longer confined to the approximately two-dimensional plane of the alloy film (which is the case with Al- and Ni–Cu-based alloys with lower melting temperatures and considerably higher thermal conductivities than the substrate material). To address the instability problem, several new substrate materials were investigated to replace a-SiN. These enabled in situ DTEM solidification studies at high temperatures typically inaccessible during in situ DTEM experiments and relevant for a broader range of alloy systems relevant for AM (the a-Al2O3 substrates used for the Ni–Cu studies represent the first success of these efforts). A second success was achieved using a new quaternary material, silicon boron carbon nitride (SiBCN), that exhibits high-temperature stability and low thermal conductivity (Zeman et al. 2010; Gentler et al. 2011) in DC magnetron sputtered thin films. These films remain amorphous at temperatures exceeding 1,600°C. Using this new substrate, membrane stability was demonstrated with a Ti-20 at.% Nb (Ti-20Nb) alloy, where melting was achieved. The melting temperature of this alloy is approximately 1,850°C, a temperature significantly higher than that achievable in conventional in situ transmission electron microscopy experiments. These amorphous SiBCN substrates offer the potential for future high-temperature in situ RS experiments using a broad class of alloys appropriate for AM.
Modeling and Simulation
The AMPE phase-field code considers thermodynamic and kinetic input data based on the Calculation of Phase Diagrams (CALPHAD) methodology (Fattebert et al. 2014) for realistic parameterization and accurate RS simulations. In this project, numerous modifications and advancements to the AMPE phase-field model (PFM) were incorporated into the code, including thermal-transport and process-parameter optimizations, and models to describe non-equilibrium RS. The thermal-transport and process-parameter optimizations include heat-capacity dependence on phase, composition, and temperature; latent heat of transformation; an equation for steady-state temperature with a heat source and temperature prescribed by specific boundary conditions; and various parameters to match the experimental laser-induced melting conditions (including energy deposited vs. time and laser shape).
The most significant accomplishment was the incorporation of a description of non-equilibrium RS. The AMPE PFM is based on the Kim–Kim–Suzuki (KKS) model (Kim et al. 1999; Kim and Kim 2001), which was designed in a thermodynamically consistent way to simulate equilibrium-phase transformations by using a chemical-potential equality constraint between the solid and liquid phases within the solid–liquid interface. By using an adjusted phase-mobility parameter (which influences the fractions of the solid and liquid phases) corresponding to RS conditions, an offset to the thermodynamic equilibrium can be generated that leads to simulation of non-equilibrium phase transformations. The drawbacks of the KKS model with the adjusted phase-mobility parameter are the computational time (the time step is decreased as the phase-mobility parameter is increased to simulate RS) and the thermodynamic description of the model. The deviation can be better described thermodynamically, using the methods of Steinbach et al (2012). This model was recently implemented in the AMPE PFM and is currently under evaluation.
Figure 2 depicts AMPE simulation results for a Ni-55Cu alloy using the KKS model with an adjusted phase-mobility parameter.
The simulations used a cooling rate of approximately 107 K/s and a thermal gradient of approximately 107 K/m, similar (i.e., same order of magnitude) to that of the DTEM RS experiments. The entire system size for the simulation was 12.8 x 12.8 µm. Figure 2(A) shows the time evolution of the temperature profile, where the heat source mimics the Gaussian laser profile of the DTEM experiments, with thermal transport and non-periodic boundary conditions activated in the AMPE code during the simulations. Figure 2(B) shows the time evolution of the phase, or solid–liquid interface, during RS. As in the DTEM experiments, the solid–liquid interface appears smooth, sharp, and morphologically planar during RS with a columnar growth morphology. Figure 2(C) shows the time evolution of the composition, with some segregation of Ni (higher Ni content) at the melt-pool perimeter, but little change with radial position along the columnar grains during RS. Figure 2(D) shows the initial (left) and final (right) simulated microstructures for comparison with the experimental microstructures (Figure 1).
Comparison of Experiment and Simulation
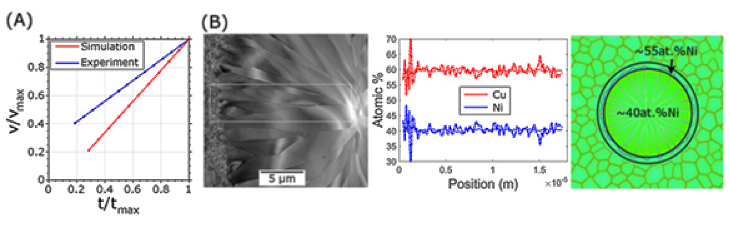
Figure 3 presents a comparison of the experimental and simulated RS results for the Ni-55Cu alloy.
Figure 3(A) presents the solid-liquid interface velocity evolution with time for both the simulated (red) and experimental (blue) RS experiments. As the melt pool dimensions and solidification times were different between the experiments and simulations (to avoid excessive computation time), the velocities and times for both data sets were normalized by their respective maxima for ease of comparison. RS microstructures will be dictated by the thermal gradient (G) and solidification-front velocity (v) (Kurz and Fisher 1984). As stated above, the thermal gradient and cooling rate were similar for the experiments and simulations. The solidification-front velocities showed excellent agreement (same order of magnitude, with absolute velocities ranging from approximately 0.3–0.9 m/s and 0.2–0.7 m/s for experiments and simulations, respectively), with a slightly higher steady-state acceleration (a) in the simulated data (asim = 1.1 m/s2 and a exp = 0.73 m/s2). The similar values of G and v yielded similar microstructures, as shown in Figure 3(B). Figure 3(B) shows (left) a high-angle annular dark-field scanning TEM (HAADF STEM) image of the re-solidified experimental melt pool. The columnar grain morphology is evident. This same columnar grain morphology is also evident in the simulated re-solidified melt pool (right). Comparisons of Figure 1(D) and Figure 2(D) also reveal the similar microstructures when comparing experiment and simulations.
The composition in the experimental melt pool was measured using energy-dispersive X-ray spectrometry (EDXS) in the TEM, and a line profile is presented in Figure 3(B, center). The region from which the line scan was acquired is shown by the white-outlined box in the HAADF STEM image of Figure 3(B). The line profile shows a fluctuation in the Ni content along the perimeter of the melt pool, with values ranging from 30 at.% Ni to approximately 55 at.% Ni. This is due to partitioning (i.e., elemental segregation) during the initial stages of re-solidification, where the solid–liquid interface is stationary (or moving very slowly) and the process is close to equilibrium. Solute (Cu) is rejected into the liquid, resulting in a Cu-rich boundary layer at the solid–liquid interface and a Ni-enriched solid phase. This is also evident in the simulated data, where the composition along the perimeter of the melt pool is approximately 55 at.% Ni. As the solid–liquid interface rapidly accelerates to steady-state columnar growth and RS conditions, large deviations from equilibrium solidification exist and the composition of the re-solidified alloy remains constant throughout the RS process. Both the experimental and simulated data show a constant composition (with no change in composition with radial position in the melt pool) of approximately 40 at.% Ni.
In summary, a comparison of the experimental and simulated RS results shows consistent interface velocities, grain morphologies, compositions, and spatial distributions of elements (Ni and Cu). Discrepancies between experimental and simulation data are due to differences in the imposed temperature profiles, leading to differences in melt-pool size and small differences in the temperature field (thermal gradient and cooling rate). Latent and specific heat were not included in the simulations, which may affect the solidification kinetics and resultant compositions. Overall, the results show significant progress toward the development of a validated predictive mesoscale microstructure evolution model for RS and AM conditions.
Impact on Mission
By developing the capability to simulate alloy solidification under RS conditions prevalent during AM, this project supports Lawrence Livermore National Laboratory's AM research. Advanced in situ materials characterization, coupled with validated predictive modeling, provides fundamental knowledge and understanding of microstructure evolution under extreme non-equilibrium conditions. This will benefit the Laboratory's AM efforts, such as the Exascale Computing Project, which is developing a multi-scale simulation framework for AM. Our work also supports the Laboratory’s core competencies in Advanced Materials and Manufacturing, and High-Performance Computing, Simulation, and Data Science, as well as its mission of nuclear weapons stockpile stewardship.
In addition, two new staff members were brought to the Laboratory through this work, strengthening its capabilities in thermodynamic modeling and materials characterization. Aurélien Perron is an expert in thermodynamic modeling and the CALPHAD methodology, and has considerable experience with phase-field simulations. John Roehling is an expert in materials characterization and DTEM.
Conclusion
The goal of this project was to develop a framework to validate predictive-modeling capabilities for microstructure evolution during rapid solidification. The outlook for this work is strong, as interest in AM continues to grow. The successes of this project have led to critical advances in the Laboratory’s AMPE phase-field code to describe rapid alloy solidification under conditions relevant to AM.
Interest in this work has led to collaborations with research groups at the Colorado School of Mines, the University of Pittsburgh, and the Oak Ridge National Laboratory. Our group continues to pursue collaborations with external organizations, including the Department of Energy and various industrial interests, such as Alcoa. This work is also part of a large, expanded multiscale modeling/experimental effort at the Laboratory to fully describe microstructure evolution in a three-dimensional AM melt pool that will both support and leverage the Exascale AM efforts.
References
Campbell, G.H., et al. 2014. “Time Resolved Electron Microscopy for in situ Experiments.” Applied Physics Reviews 1:041101. doi: 10.1063/1.4900509.
Dorr, M.R., et al. 2010. “A Numerical Algorithm for the Solution of a Phase-Field Model of Polycrystalline Materials.” Journal of Computational Physics 229: 626—641. doi: 10.1016/j.jcp.2009.09.041.
Fattebert, J.-L., et al. 2014. “Phase-Field Modeling of Coring During Solidification of Au-Ni Alloy Using Quaternions and CALPHAD Input.” Acta Materialia 62: 89—104. doi: 10.1016/j.actamat.2013.09.036.
Gengler, J.J., et al. 2011. “Thermal Conductivity of High-Temperature Si-B-C-N Thin Films.” Surface & Coatings Technology 206: 2030—2033. doi: 10.1016/j.surfcoat.2011.07.058.
Gill, S.C., and W. Kurz. 1993. “Rapidly Solidified Al-Cu Alloys-I. Experimental Determination of the Microstructure Map.” Acta Metallurgica et Materialia 41 (12): 3563—3573. doi: 10.1016/0956-7151(93)90237-M.
Herlach, D.M. 1994. “Non-Equilibrium Solidification of Undercooled Metallic Melts.” Materials Science and Engineering: Reports 4: 177—272. doi: 10.1016/0927-796X(94)90011-6.
Kim, S.G., and W.T. Kim. 2001. “Phase-Field Model of Rapid Solidification.” Materials Science and Engineering A 304-306: 282—286. doi: 10.1016/S0921-5093(00)01453-2.
Kim, S.G., et al. 1999. “Phase-Field Model for Binary Alloys.” Physical Review E 60 (6): 7186—7197. doi: 10.1103/PhysRevE.60.7186.
King, W.E. 2014. “Advancing Metal AM at its Most Fundamental Level.” Journal of Materials (JOM) 66 (11): 2202—2203. doi: 10.1007/s11837-014-1166-x.
Kittl, J.A., et al. 2000. “Complete Experimental Test of Kinetic Models for Rapid Alloy Solidification.” Acta Materialia 48 (20): 4797—4811. doi: 10.1016/S1359-6454(00)00276-7.
Kline, J.E., and J.P. Leonard. 2005. “Rapid Lateral Solidification of Pure Cu and Au Thin Films Encapsulated in SiO 2.” Applied Physics Letters 86 (20): 201902. doi: 10.1063/1.1925784.
Kulovits, A., et al. 2009. “Electron Microscopy of Geometrically Confined Copper Thin Films After Rapid Lateral solidification.” Thin Solid Films 517 (13): 3629—3634. doi: 10.1016/j.tsf.2008.11.132.
——— 2011. “Revealing the Transient States of Rapid Solidification in Aluminum Thin Films Using Ultrafast in situ Transmission Electron Microscopy.” Philosophical Magazine Letters 91 (4): 287—296. doi: 10.1080/09500839.2011.558030.
Kurz, W., and D.J. Fisher. 1984. Fundamentals of Solidification. Rockport, MA: Trans Tech Publications.
Kurz, W., and P. Gilgien. 1994. “Selection of Microstructures in Rapid Solidification Processing.” Materials Science and Engineering A 178 (1—2): 171—178. doi: 10.1016/0921-5093(94)90538-X.
LaGrange, T., et al. 2015. “Movie-Mode Dynamic Electron Microscopy.” MRS Bulletin 40: 22—28. doi: 10.1557/mrs.2014.282.
Mani, M., et al. 2015. “NISTIR 8036 - Measurement Science Needs for Real-Time Control of Additive Manufacturing Powder Bed Fusion Processes.” National Institute of Standards and Technology. doi: 10.6028/NIST.IR.8036.
McKeown, J.T., et al. 2014. “In situ Transmission Electron Microscopy of Crystal Growth-Mode Transitions During Rapid Solidification of a Hypoeutectic Al-Cu Alloy.” Acta Materialia 65: 56—68. doi: 10.1016/j.actamat.2013.11.046.
McKeown, J.T., et al. 2016. “Time-Resolved in situ Measurements During Rapid Alloy Solidification: Experimental Insight for Additive Manufacturing.” Journal of Materials (JOM) 68 (3): 985—999. doi: 10.1007/s11837-016-1842-0.
Rasband, W.S. 2018. “ImageJ: Image Processing and Analysis in Java.” U.S. National Institutes of Health. Accessed July 19. http://imagej.nih.gov/ij/.
Schneider, C.A., et al. 2012. “NIH Image to ImageJ: 25 Years of Image Analysis.” Nature Methods 9: 671—675. doi: 10.1038/nmeth.2089.
Steinbach, I., et al. 2012. “Phase-Field Model with Finite Interface Dissipation.” Acta Materialia 60 (6—7): 2689—2701. doi: 10.1016/j.actamat.2012.01.035.
Zeman, P., et al. 2010. “Thermal Stability of Magnetron Sputtered Si-B-C-N Materials at Temperatures up to 1700°C.” Thin Solid Films (1): 306—311. doi: 10.1016/j.tsf.2010.08.080.
Zhong, R., et al. 2009. “Four-Zone Solidification Microstructure Formed by Laser Melting of Copper Thin Films.” Applied Surface Science 256 (1): 105—111. doi: 10.1016/j.apsusc.2009.07.084.
Zimmermann, M., et al. 1989. “Rapid Solidification of Al-Cu Eutectic Alloy by Laser Remelting.” Acta Metallurgica 37 (12): 3305—3313. doi: 10.1016/0001-6160(89)90203-4.
Publications and Presentations
Egan, G.C., et al. 2018. “In situ TEM characterization of Unsteady Crystallization During Laser Processing of Amorphous Germanium.” Acta Materialia 143: 13—19. LLNL-JRNL-733656.
Fattebert, J.-L., et al. 2016. “Coupling CALPHAD to Phase-Field Modeling: A Pathway to the Prediction of Microstructures in Additive Manufacturing.” The Minerals, Metals and Materials Society (TMS) Annual Conference, Nashville, TN, February 2016. LLNL-PRES-683459.
McKeown, J.T., et al. 2015a. “Time-Resolved in situ Characterization of Laser-Induced Rapid Solidification.” The Minerals, Metals and Materials Society (TMS) Annual Conference, Orlando, FL, March 2015. LLNL-PRES-668599.
——— 2015b. “Experiments and Simulations of Phase Transformations at Similar Length and Time Scales.” Mach 2015, Annapolis, MD, April 2015. LLNL-PRES-669186.
McKeown, J.T., et al. 2016a. “Toward Predicting Rapidly Solidified Microstructures of Metallic Alloys.” Materials Science & Technology (MS&T) Conference, Salt Lake City, UT, October 2016. LLNL-PRES-705779.
——— 2016b. “Microstructure Evolution During Laser-Induced Rapid Alloy Solidification.” The Minerals, Metals and Materials Society (TMS) Conference, Nashville, TN, February 2016. LLNL-PRES-682701.
——— 2017. "Microstructure Evolution During Laser-Induced Rapid Alloy Solidification.” Recent Advances in Integrated Computational and Experimental Methods for Additive Manufacturing, Golden, CO, September 2017. LLNL-PRES-737898.
Perron, A., et al. Forthcoming. “Matching Time and Spatial Resolutions of Rapid Solidification: Dynamic TEM Experiments Coupled to CALPHAD-Informed Phase-Field Simulations.” Modeling and Simulation in Materials Science and Engineering. LLNL-JRNL-727840-DRAFT.
Perron, J. et al. 2016. “Solidification Across the Multi-Scale Landscape." 8th International Conference on Multiscale Materials Modeling, Dijon, France, October 2016. LLNL-PROP-677182.
Roehling, J.D., et al. 2015a. “In situ Characterization and Microstructure Modeling of Rapid Alloy Solidification.” Advanced Qualification of Additive Manufacturing Materials (AM) Workshop, Santa Fe, NM, July 2015. LLNL-POST-674472.
——— 2015b. “Imaging the Rapid Solidification of Metallic Alloys in the TEM.” Microscopy & Microanalysis (M&M), Portland, OR, August 2015. LLNL-PRES-675759.
——— 2015c. “Monitoring Rapid Solidification of Metallic Alloys in the TEM.” Materials Science & Technology (MS&T), Columbus, OH, October 2015. LLNL-PRES-677781.
Roehling, J.D., et al. 2017a. “Progress Toward Predicting Rapidly Solidified Microstructures of Metallic Alloys.” The Minerals, Metals and Materials Society (TMS) Conference, San Diego, CA, February 2017. LLNL-POST-725018.
——— 2017b. “Rapid Solidification Growth Mode Transitions in Al-Si Alloys by Dynamic Transmission Electron Microscopy.” Acta Materialia 131: 22—30. LLNL-JRNL-728617.
——— 2017c. “Modulating Laser Intensity Profile Ellipticity for Microstructural Control During Metal Additive Manufacturing.” Acta Materialia 128: 197—206. LLNL-JRNL-713205.
Turchi, P.E.A. 2015. “Ab initio Quantum Mechanics-Aided Thermodynamics of Materials.” DFG-sponsored “Early Career Investigators” Workshop, Schlitz, Germany, June 2015. LLNL-PRES-671202.
Turchi, P.E.A., et al. 2015. “Is Alloy Thermodynamics Still a Matter of Principles?” The Minerals, Metals and Materials Society (TMS) Conference, Orlando, FL, March 2015. LLNL-ABS-656223.
Turchi, P.E.A. 2016a. “Microstructure Modeling in Support of Additive Manufacturing.” Department of Materials Engineering, Federal Institute of Materials Research and Testing (BAM), Berlin, Germany, January 2016. LLNL-PRES-680801.
——— 2016b. “Old Time - Present - Future of Computational Materials Science.” Department of Materials Engineering, Federal Institute of Materials Research and Testing (BAM), Berlin, Germany, January 2016. LLNL-PRES-680741.
——— 2016c. “Why Is Alloy Theory Still a Matter of Principles?” Materials Science and Engineering (MSE) Conference, Darmstadt, Germany, September 2016. LLNL-PRES-703542.
Turchi, P.E.A. 2017. “Density Functional Theory and Thermodynamics Applied to Complex Materials - Is There Still a Need for ab initio-Aided Alloy Theory?” Physics Department, University of Rennes, Rennes, France, June 2017. LLNL-PRES-709482.