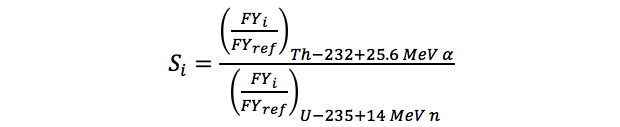Narek Gharibyan (16-FS-002)
Abstract
Relative fission yield measurements were made for 50 fission products from 25.6 ± 0.5-MeV alpha-induced fission of thorium-232. Quantitative comparison of these experimentally measured fission yields with the evaluated fission yields from 14-MeV neutron-induced fission of uranium-235 demonstrates the feasibility of the proposed fission-proxy method. This new technique is based on the Bohr-independence hypothesis, which postulates that the process of formation and decay are completely independent of each other. This technique permits the measurement of fission yields from an alternate reaction pathway: thorium-232 plus an 25.6-MeV alpha particle yielding uranium-236 versus uranium-235 plus a 14-MeV neutron yielding uranium-236. This measurement is made possible given that the fission process associated with the same compound nucleus is independent of its formation. Other suitable systems that can potentially be investigated in this manner include but are not limited to plutonium-239 and uranium-237.
Background and Research Objectives
The radiochemical measurement of a fission yield from a nuclear event depends on the fidelity of the cumulative fission yields—that is, the number of atoms per 100 fissions of a nuclide produced directly and through its precursors. The measured radioactivities of the fission products from nuclear debris can be converted to a number of fissions, using the best-available cumulative fission yields obtained from the fraction of the total debris yield for a given sample. This bomb fraction is typically extracted from tracer packages placed around the device. A total yield can be directly measured through radiochemical methods that rely on the knowledge of the bomb fraction and cumulative fission product yields. In addition to a total yield, cumulative fission yields can provide information regarding the composition of a device's nuclear fuel. The direct measurements of the cumulative fission yields with 14-MeV neutrons in traditional accelerator facilities are unavoidably accompanied by down-scattered, low-energy neutrons. These low-energy neutrons are more effective than 14-MeV neutrons at inducing fission in fissile materials, thus affecting the accuracy of cumulative fission-yield measurements. As a result, the cumulative fission yields for fissile materials have the highest associated uncertainties for 14-MeV neutrons compared to fission spectrum and thermal neutrons and compared to 14-MeV neutron fission yields from non-fissile materials.1,2
Our work focused on determining the feasibility of a novel method for measuring 14-MeV neutron fission yields without using neutrons. This method uses a fission-proxy reaction method—an indirect method for creating the same compound nucleus as that produced from 14-MeV neutrons on fissile isotopes. This fission-proxy reaction method, which has a significant advantage compared to the direct measurements with neutrons, is based on the Bohr independence hypothesis. This hypothesis states, in essence, the “only memory that the system (the compound nucleus undergoing fission) maintains is that of the constants of the motion: momentum, angular momentum, number of nucleons, parity, energy and isotopic spin.”3 In other words, once the compound nucleus is formed, it proceeds to decay into its reaction products independent of its formation process.
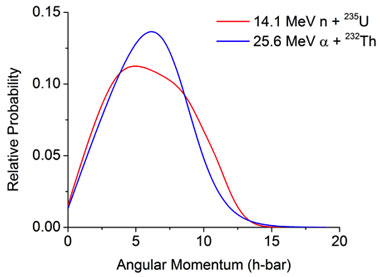
Validating this fission-proxy method involved measuring fission yields from 25.6-MeV alpha-induced fission of thorium-232, which simulates the same excited compound nucleus (uranium-236, 20.6 MeV) as that produced from 14-MeV neutrons on uranium-235. Initial calculations indicated a large overlap in the angular momentum distribution for this compound nucleus from both reactions (Figure 1). Therefore, the nucleus is expected to undergo fission in the same manner, independent of the production reaction pathway. The 14-MeV neutron fission yields for uranium-235 are well-characterized, whereas the proposed proxy reaction fission yields, thorium-232 plus a 25.6-MeV alpha particle, were not well known because there are no evaluated fission yields.2
The alpha-induced fission yields for thorium-232 in the literature are very limited, having been measured prior to the major developments in high-resolution gamma-ray detectors.4–9 Hence, these measurements were made strictly through beta counting following extensive radiochemistry separation procedures. Given the inadequate literature data for fission yields from thorium-232 plus a 25.6-MeV alpha particle, it was difficult to assess the feasibility of the fission-proxy method without significant improvement of the available fission yields.
Our objective was to investigate the feasibility of the fission-proxy method by performing a detailed comparison of the fission yields from the two different reactions: thorium-232 plus a 25.6-MeV alpha particle versus uranium-235 plus a 14-MeV neutron. Because the literature data for the thorium-232 system is limited, a series of alpha-induced fission yield measurements on thorium-232 were performed using the Tandem Van de Graaff accelerator located at LLNL's Center for Accelerator Mass Spectrometry, along with the use of Lawrence Livermore's radiochemistry laboratories and the Nuclear Counting Facility. These experimentally measured fission yields were then compared to the evaluated fission yields for 14-MeV neutrons on uranium-235 to assess the fidelity of the fission-proxy method.2 The original proposal had outlined fission yield measurements for 45 fission products, but we actually measured a total of 50 fission product yields. The results, summarized in the next section, indicated that the proposed fission-proxy method is a suitable technique for measuring 14-MeV neutron fission yields through an appropriate alternate-reaction pathway. As such, it appears that the application of the Bohr-independence hypothesis for the measurement of fission yields is acceptable.
Scientific Approach and Accomplishments
Fission yield measurements were performed by irradiating thorium-232 foils with alpha particles in a foil stack configuration that included aluminum catcher foils to ensure the complete collection of the fission products from recoil. Three irradiation experiments were conducted at the Center for Accelerator Mass Spectrometry facility. The first experiment, CAMS-N160331, utilized a thick thorium foil (28 mg/cm2), where the beam energy loss through the foil was significant at 2.6 MeV. Additionally, the thickness of catcher foils (2.2 mg/cm2), were not sufficient to stop the recoil of the fission products. Thus, the results from the first experiment were not included in the final fission yield calculations, but formed the basis of the final experimental design utilized for the next two irradiations, including radiation counting methods.
The second experiment, CAMS-N160523, used a thin thorium foil (11 mg/cm2, 99.5% purity) with 4.3-mg/cm2 and 5.8-mg/cm2 aluminum catcher foils, front and back, respectively. The relative beam intensity on target was monitored throughout the 5.5 h of beam-on-target at approximately 90-pnA current. Based on stopping-power tables generated through the SRIM module (a subroutine used for applications that require ion stopping powers or ranges), the alpha beam energy through the thorium foil was calculated to be 25.6 ± 0.5 MeV, with the uncertainty corresponding to the energy loss through the foil.10 This calculation assumes incident beam energy is accurate within 10 keV, which is a conservative estimate for Van de Graaff accelerators, and does not take into consideration energy straggling through the foil stack. Following the irradiation, the thorium foil along with its catcher foils were dissolved, and an aliquot was taken for direct counting for analysis of the high-yield refractory fission products. Separate radiochemical procedures were performed on the remaining solution to quantify low-yield fission products of interest: cadmium, silver, and lanthanides. The last set of irradiation experiments, CAMS-N160819, which comprised two irradiation sessions, mirrored the experimental setup of CAMS-N160523. This last set resulted in a whole thorium foil sample for direct counting and chemical fractions of zinc, niobium, and palladium.
Irradiated foils and chemical fractions were counted on coaxial and planar high-purity germanium detectors. Gamma-ray spectral evaluation was performed with the GAMANAL code (Code System for Computerized Quantitative Analysis by Gamma-Ray Spectrometry).11 When possible, decay curve analysis was utilized to further ensure the validity of the fission product measurement. The uncertainties associated with the measured fission yields (cumulative and independent/shielded) are one-sigma and include counting statistics, uncertainty in the half-life, and photon intensities.12,13 The final data set of measured relative fission yields (55 measurements covering 50 different fission products) for thorium-232 plus a 25.6-MeV alpha particle is presented in Table 1.
Table 1. Measured relative fission yields for thorium-232 plus a 25.6 ± 0.5-MeV alpha particle.
| Fission product | Reference | Fission yield (relative to reference) | % Error | Type* |
| Zn-72 | Sr-91 | 0.00249 | 18.1 | c |
| Sr-91 | Zr-97 | 0.901 | 7.0 | c |
| Y-93 | Zr-97 | 1.10 | 13.9 | c |
| Y-93 | Ce-141 | 1.13 | 15.2 | c |
| Zr-95 | Zr-97 | 1.05 | 0.5 | c |
| Nb-96 | Sr-91 | 0.000762 | 10.7 | s |
| Mo-99 | Zr-97 | 1.001 | 2.0 | c |
| Ru-103 | Zr-97 | 0.642 | 1.2 | c |
| Ru-105 | Ru-103 | 0.645 | 1.3 | c |
| Rh-105 | Zr-97 | 0.455 | 2.5 | c |
| Ru-106 | Ru-103 | 0.593 | 9.1 | c |
| Ag-111 | Zr-97 | 0.265 | 4.7 | c |
| Pd-111m | Pd-109 | 0.0138 | 23.1 | c* |
| Pd-112 | Pd-109 | 1.14 | 15.3 | c |
| Ag-113 | Ag-111 | 1.25 | 6.9 | c |
| Cd-115m | Cd-115 | 0.627 | 45.2 | c* |
| Cd-117 | Cd-115 | 0.591 | 4.0 | c |
| Cd-117m | Cd-115 | 0.217 | 4.1 | c* |
| Sb-124 | Zr-97 | 0.00716 | 5.1 | s |
| Sn-125 | Zr-97 | 0.181 | 30.0 | c |
| Sb-126 | Zr-97 | 0.0596 | 2.2 | s |
| Sb-127 | Zr-97 | 0.388 | 2.5 | c |
| Sb-128 | Zr-97 | 0.174 | 5.5 | c |
| Te-129m | Zr-97 | 0.164 | 36.2 | c* |
| I-131 | Zr-97 | 0.964 | 1.0 | c |
| Te-131m | Zr-97 | 0.282 | 3.5 | c* |
| Te-132 | Zr-97 | 0.831 | 4.0 | c |
| I-133 | Zr-97 | 1.15 | 3.1 | c |
| Xe-133 | Zr-97 | 0.0751 | 35.4 | i |
| Cs-134 | Cs-136 | 0.280 | 29.0 | s |
| Cs-136 | Zr-97 | 0.0558 | 5.2 | s |
| Cs-136 | Cs-137 | 0.0523 | 6.3 | s |
| Cs-137 | Zr-97 | 1.05 | 3.3 | c |
| Ba-140 | Zr-97 | 0.877 | 1.1 | c |
| La-140 | Zr-97 | 0.0126 | 9.0 | i |
| Ce-141 | Zr-97 | 0.870 | 0.9 | c |
| La-142 | Ce-141 | 1.00 | 7.5 | c |
| Ce-143 | Zr-97 | 0.713 | 1.3 | c |
| Ce-143 | Ce-141 | 0.849 | 3.4 | c |
| Ce-144 | Ce-141 | 0.693 | 4.0 | c |
| Pr-145 | Ce-141 | 0.531 | 3.9 | c |
| Nd-147 | Zr-97 | 0.312 | 4.4 | c |
| Nd-147 | Ce-141 | 0.373 | 4.1 | c |
| Nd-149 | Ce-141 | 0.214 | 10.0 | c |
| Pm-149 | Ce-141 | 0.194 | 7.0 | c |
| Pm-151 | Zr-97 | 0.0850 | 5.6 | c |
| Pm-151 | Ce-141 | 0.103 | 4.8 | c |
| Sm-153 | Ce-141 | 0.0493 | 2.7 | c |
| Eu-155 | Ce-141 | 0.0175 | 19.1 | c |
| Sm-156 | Ce-141 | 0.0169 | 16.2 | c |
| Eu-156 | Zr-97 | 0.0123 | 10.3 | c |
| Eu-156 | Ce-141 | 0.0145 | 9.3 | c |
| Eu-157 | Ce-141 | 0.00950 | 19.1 | c |
| Gd-159 | Ce-141 | 0.00419 | 30.1 | c |
| Tb-161 | Ce-141 | 0.00255 | 6.8 | c |
*Types include c = cumulative, c* = isomer/cumulative, s = shielded, i = independent (ingrowth correction from parent).
Qualitative comparison of these relative fission yields (normalized to zirconium-97) to those from uranium-235 plus a 14-MeV neutron are presented in Figure 2. The similarities between the measured cumulative fission yields (black circles) and the uranium-235 literature data (gray line) is obvious, but to provide a more quantitative comparison, the same data set of fission yields (FY) for thorium-232 (Th-232) plus a 25.6-MeV alpha particle (α) and uranium-235 (U-235) plus a 14-MeV neutron (n) is converted to a ratio of ratios, the S-value shown in Equation 1.
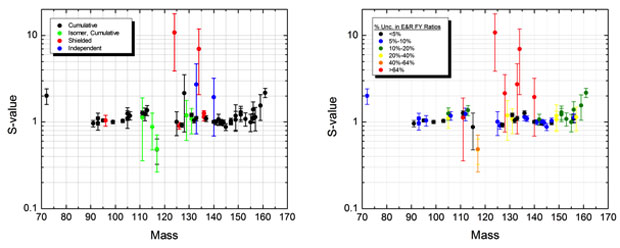
If the fission yields from both systems are in agreement, the population distribution of the S-value from the 55 measurements (Table 1) should be centered on unity, as is evident in Figure 3. Although this further confirms the similarities in the fission yields, it does not demonstrate the impact of the uncertainties associated with the experimental measurements and the evaluated fission yields. Thus, the distribution from the individual measurements is provided in Figure 4. The plot on the left is color-coded for the type of fission yield, where those furthest from unity are either shielded or isomer fission yields with large associated uncertainties. The plot on the right is color-coded to demonstrate the uncertainties associated with the evaluated fission yields from uranium-235 plus a 14-MeV neutron. The fission yield measurements with S-values furthest from unity correspond to those with the largest uncertainties in the literature data. Lastly, a reduced chi-squared of 2.4 was calculated for these 55 measurements as compared to the uranium-235 literature fission yields. If the extremely poor fission yield measurements from literature (those with >64% uncertainties) are excluded from consideration, a reduced chi-squared of 1.3 is obtained for 49 fission yield measurements.
Therefore, given the difficulties associated with measuring of fission yields from extremely complex gamma-ray spectra in addition to the large uncertainties in both experimental and literature data, the agreement between the fission yields from these two systems is significant. Although repeating some of these measurements for stability valley fission products palladium, silver, and cadmium, and for the lightest/heaviest fission products zinc and terbium is necessary to confirm the few discrepancies, the measured fission yield set as a whole clearly supports the application of the compound-nucleus “fission-proxy” model for the measurement of fission yields.
Impact on Mission
Our study directly supported the nuclear, chemical, and isotopic science and technology core competency—the work required radiochemical separation developments, nuclear detection and counting, and extensive data analysis. Furthermore, by demonstrating the feasibility of the fission-proxy method, fission yield measurements for difficult-to-study systems relevant to stockpile stewardship are now possible, such as for plutonium-239 and uranium-237, which have identified, proper fission-proxy reactions. Furthermore, applications of the fission-proxy method for the production of fission products that mimic unique fission product distributions are of interest to programs in support of realistic post-detonation debris production and validation of such forensic debris data.
Conclusion
We have successfully demonstrated the feasibility of the proposed fission-proxy method. It will allow for the fission yield measurements of more difficult-to-study systems where the unavailability of target material, such as uranium-237, makes direct measurements with neutron-based experiments impossible. We plan to reach out to both external and Livermore internal programs for support of continued research and development of the fission-proxy method.
References
- Nethaway, D. R., PROPHET fission yields. LLNL Nuclear Chemistry Division Memorandum. Lawrence Livermore National Laboratory, Livermore, CA (1985). LJW-45-85-189.
- England, T. R., and B. F. Rider, Evaluation and compilation of fission product yields. Los Alamos National Laboratory, Los Alamos, NM (1993). LA-UR-94-3106.
- Fluss, M. J., et. al., "Investigation of the Bohr-Independence hypothesis for nuclear reactions in the continuum: α+Co59, p+Ni62 and α+Fe56, p+Co59." Phys. Rev. C 187(4), 1449 (1969). https://doi.org/10.1103/PhysRev.187.1449
- Foreman, B. M., et. al., "Spallation-fission competition in heavy-element reactions: Th232+He4 and U233+d." Phys. Rev. 116(2), 382 (1959). https://doi.org/10.1103/PhysRev.116.382
- Hicks, H. G., et. al., "Further radiochemical studies of fission of the U236* compound nucleus." Phys. Rev., 128, 700 (1962). https://doi.org/10.1103/PhysRev.128.700
- Ford, G. P., and R. B. Leachman, "Fission mass yield dependence on angular momentum." Phys. Rev. 137, B826 (1965). https://doi.org/10.1103/PhysRev.137.B826
- Nethaway, D. R., and H. B. Levy, "Effect of increasing excitation energy on nuclear charge distribution in fission." Phys. Rev. 139, B1505 (1965). https://doi.org/10.1103/PhysRev.139.B1505
- McHugh, J. A., and M. C. Michel, "Fission-fragment mass and charge distribution for the moderately excited U236 compound nucleus." Phys. Rev. 172, 1160 (1968). https://doi.org/10.1103/PhysRev.172.1160
- Eberth, J., and J. Simpson, "From Ge(Li) detectors to gamma-ray tracking arrays—50 years of gamma spectroscopy with germanium detectors." Progr. Part. Nucl. Phys. 60(2), 283 (2008). http://dx.doi.org/10.1016/j.ppnp.2007.09.001
- Ziegler, J. F., M. D. Ziegler, and J. P. Biersack, "SRIM—The stopping and range of ions in matter." Nucl. Instrum. Meth. Phys. Res. B 268(11–12), 1818 (2010). http://dx.doi.org/10.1016/j.nimb.2010.02.091
- Gunnik, R., and J. Niday, Computerized quantitative analysis of gamma-ray spectrometry. Lawrence Livermore National Laboratory, Livermore, CA (1972). UCRL-51061.
- National Nuclear Data Center, Nuclear science references. Brookhaven National Laboratory, Upton, New York (2016). http://www.nndc.bnl.gov/nsr
- Firestone, R. B., and V. S. Shirley, Table of isotopes, 8th edition. John Wiley & Sons, Inc., San Francisco, CA (1996).